Question on inscribed triangles - and on outer triangles.
Given a planar convex body C, we can define 2 inscribed triangles:
T_a - inscribed triangle of maximum area
T_r - inscribed triangle with largest incircle
Question 1: Are these 2 triangles different from each other for a general C? In cases where the optimal triangles are different, one can try to quantify the difference.
Question 2: Corresponding to these 2 contained triangles, we can think of 2 containing triangles that minimize area and inradius and ask whether those 2 external triangles are distinct in general.
Note 2: The inscribed (containing) triangle with largest (smallest) area is also the inscribed triangle with the largest area Steiner in-ellipse (smallest area Steiner-circumellipse).
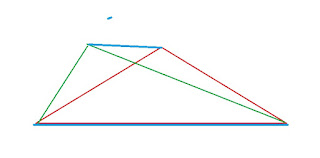
Answer to Question 1:
Consider the quadrilateral above. The top left vertex is very slightly higher than the top right vertex - and these two vertices have a substantial x separation. So, the green triangle is the largest area triangle inscribed in the quad (by a small margin) and the red triangle which is isosceles has the largest incircle (note that the inradius of a triangle = area / semiperimeter). This means answer to question 1 above is "the two triangles need not be identical".
Guess on question 2:
Consider a hexagon formed by the itnersection of the two triangles in answer 1 (one of them an isosceles). Then for this hexagon, the smallest area containing triangle would be isosceles one and the other triangle would be another container with slightly larger area but with smaller incircle.
Note: The Steiner circumellipse of a triangle is the containing ellipse with least area but it is not usually the containing ellipse with least perimeter. This follows from the center of the Steiner ellipse being the centroid of the triangle - for a thin and long isosceles triangle, the containing ellipse with least perimeter will have its center close to the mid point of the altitude of the triangle thru the apex and not the centroid. That brings up the question of how to find the that container for a triangle.



0 Comments:
Post a Comment
<< Home